The "No Shortcuts" Paradox

by Thomas Kühne

The Ancient Fallacy

The world has long been fooled by a certain Mr. Pythagoras whose Pythagorean theorem (c²=a²+b², where c is the length of the hypotenuse in a right triangle and a & b are the lengths of the two legs) can be used to infer that the shortest distance s = √((x₂-x₁)²+(y₂-y₁)²) (also called the Euclidean distance) between two points P₁=(x₁,y₁) & P₂=(x₂,y₂) is shorter than the Manhattan distance m = h + v, where h = |x₂-x₁| and v = |y₂-y₁|, between the same two points.

The Truth

The above suggests that the shortest distance s represents a shortcut in comparison to the path constructed by following |P₁P| (h = |x₂-x₁|) and then |PP₂| (v = |y₂-y₁|). However, nothing could be further from the truth. Apart from empirical results that show that shortcuts even result in prolonged traveling, it can be mathematically proven that the alleged shortcut |P₁P₂| with length s is exactly as long as the Manhattan distance h + v.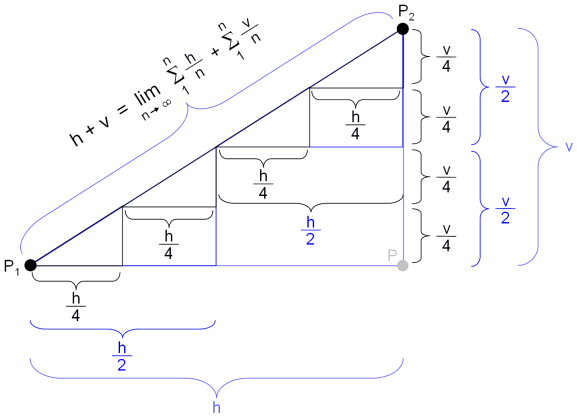

The Proof

Consider the above figure. The horizontal distance between P₁ & P₂ is h and the vertical distance v. The total distance between P₁ and P₂ via P hence is h + v. Let us start approximating the straight line between P₁ and P₂ by replacing the large triangle P₁, P, P₂ with two triangles where the legs have length h/2 and v/2 correspondingly (see above). A better approximation is obtained by using four triangles with leg lengths h/4 and v/4. The more triangles we use, the better the triangles will approximate the straight line between P₁ and P₂. In the limit, the leg lengths will be zero and the infinite number of triangles will match the straight line between P₁ and P₂. However, note that the sum of the horizontal distances — independently of the granularity of the division — is always h and the sum of the vertical distances is always v. As a result, the length of s is h + v as opposed to √(h²+v²) as commonly believed. Consequently, it does not matter whether one proceeds from P₁ to P₂ following a straight line or via P, the length of the path is always h + v. In other words, there are no shortcuts.

The Fun Part

Hint: As is typical for a falsidical paradox, the above rationalization suggests a result that is not only counterintuitive but also wrong. The fun part is in figuring out where the flaw in the apparently logical reasoning is.

Spoiler: Think about in what way the breaking up into small horizontal and vertical stretches constrains a particle moving along them.